Zeno's Paradoxes are a set of philosophical problems proposed by the ancient Greek philosopher Zeno of Elea in the 5th century BCE. These paradoxes aim to challenge our understanding of motion, space, and time by presenting seemingly contradictory scenarios. Although they were initially intended to support the philosophical concept of Parmenidean monism, they have sparked debates and discussions among mathematicians, physicists, and philosophers for centuries.

 1. Dichotomy Paradox: This paradox argues that motion is impossible since an object must first cover half the distance to its destination, then half of the remaining distance, and so on, resulting in an infinite number of steps. Therefore, according to Zeno, an object can never reach its destination. For example, if you want to walk to the other side of a room, you first have to cover half the distance, then half of the remaining distance, and so on, leading to an infinite number of steps.

 2. Achilles and the Tortoise Paradox: This paradox presents a scenario where Achilles, a great Greek hero, races against a tortoise. However, the tortoise is given a head start. Zeno argues that Achilles can never overtake the tortoise since, by the time Achilles reaches the tortoise's starting point, the tortoise would have moved a bit further. Again, this paradox suggests that motion is impossible. However, in reality, Achilles would indeed overtake the tortoise because we can mathematically calculate the time it would take for Achilles to reach the tortoise's starting point and surpass it.

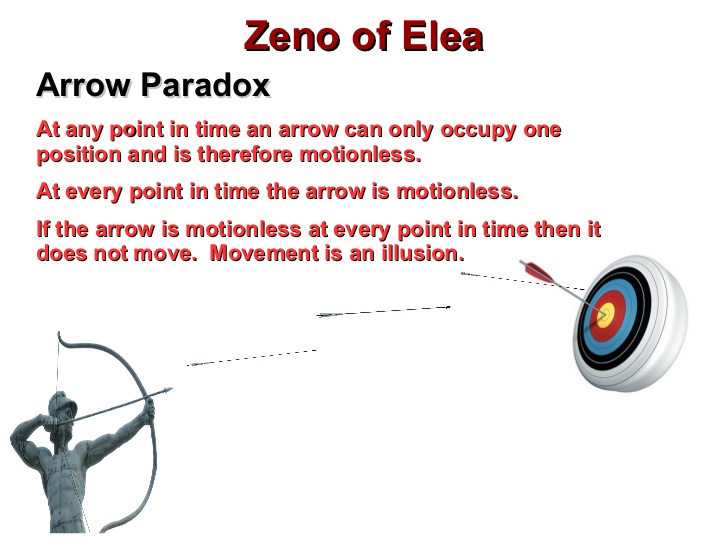
 3. Arrow Paradox: Zeno's Arrow Paradox claims that an arrow in flight is motionless at any given instant. Zeno argues that at any specific moment, the arrow occupies a single position, and therefore, it must be at rest. However, since motion is the result of a series of these instants, Zeno concludes that motion is an illusion. This paradox challenges our understanding of time and the concept of continuous motion. However, modern physics and calculus provide a mathematical framework to describe motion by considering infinitesimal time intervals.

 It is important to note that Zeno's Paradoxes were presented in the form of paradoxes to provoke philosophical discussions rather than to provide definitive answers. Over time, mathematicians and philosophers have developed various solutions and interpretations to address these paradoxes. For example, mathematicians have used the concept of limits, calculus, and mathematical series to resolve these paradoxes. Philosophers have also proposed different interpretations, such as the idea that Zeno's paradoxes highlight the limitations of our intuitive understanding of motion and the need for more sophisticated mathematical models.

 References:
 1. Zeno's Paradoxes. (2021). Stanford Encyclopedia of Philosophy. https://plato.stanford.edu/archives/win2016/entries/paradox-zeno/
 2. Zeno's Paradoxes. (2021). Internet Encyclopedia of Philosophy. https://www.iep.utm.edu/zeno-par/